

It also formulates Branchistochrone problem. It also used to find the curve between two given points in the plane that yields a surface of revolution around a given axis. geodesic) between two given points on a surface. The mathematical techniques developed to solve this type of problem are collectively known as the calculus of variations. Our first example of a variational problem is the planar geodesic: given two points lying in a. The calculus of variations gives us precise analytical techniques to find the shortest path (i.e. If there are no constraints, the solution is a straight line. For the connection on the target manifold, we get the expected result that it is a metric connection. A simple example of such a problem is to find the curve of shortest length connecting two points. One example is finding the curve giving the shortest distance between two points - a straight line, of course, in Cartesian geometry (but can you prove it?) but less obvious if the two points lie on a curved surface (the problem of finding geodesics.) Using the well-established formalism of calculus of variations on fibred manifolds, we solve the weak inverse problem for the equation of geodesic mappings and get a variational equation, which is a consequence of the geodesic mappings equation. If the Earth is treated as a sphere, the geodesics are great circles (all of which are closed. The solution of a triangulation network on an ellipsoid is therefore a set of exercises in spheroidal trigonometry ( Euler 1755 ).
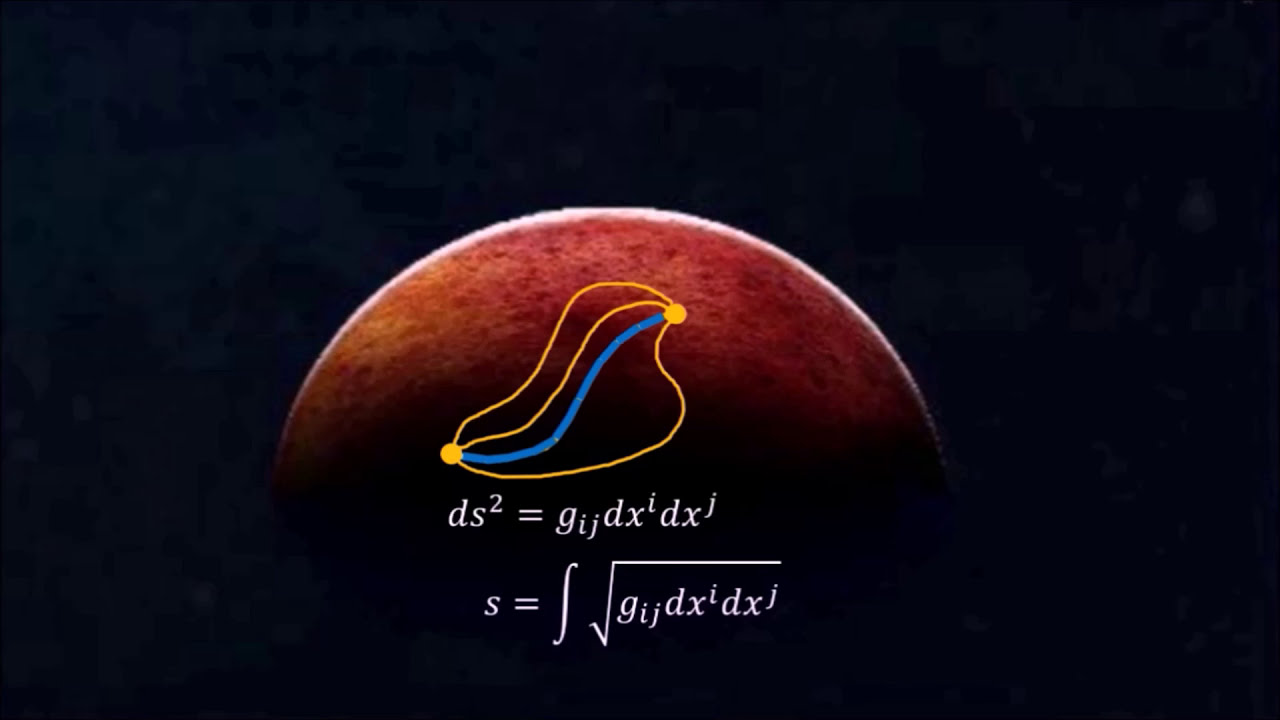
Many problems involve finding a function that maximizes or minimizes an integral expression. A geodesic is the shortest path between two points on a curved surface, analogous to a straight line on a plane surface. MATH0043 Handout: Fundamental lemma of the calculus of variations.The Euler-Lagrange Equation, or Euler’s Equation.MATH0043 §2: Calculus of Variations MATH0043 §2: Calculus of Variations


 0 kommentar(er)
0 kommentar(er)
